In Exercises 5 and 6, use the scatterplot to find the value of...

Question

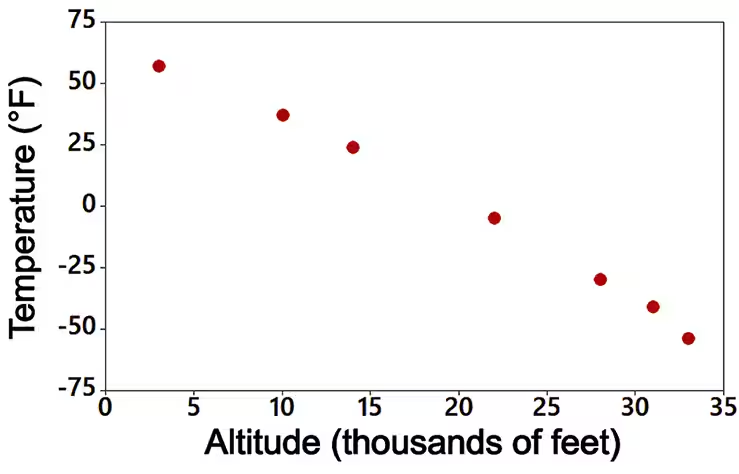
In Exercises 5 and 6, use the scatterplot to find the value of the rank correlation coefficient and the critical values corresponding to a 0.05 significance level used to test the null hypothesis of . Determine whether there is a correlation.

Altitude and Temperature Shown below is a scatterplot of altitudes (thousands of feet) and outside air temperatures (degrees Fahrenheit) recorded by the author during a Delta flight from New Orleans to Atlanta.

Scatterplot showing negative correlation between altitude (thousands of feet) and temperature (°F) during a flight.

Accepted Answer

Hello everyone. Let's take a look at this question together. Shown below is a scatter plot of resting heart rates in beats per minute and reaction times in milliseconds measured in a group of participants during a cognitive performance test. And here we have our scatter plot of the reaction times in milliseconds and the resting heart rate in BPM. Find the value of the rank correlation coefficient or Spearman's row. Find the critical values corresponding to a 0.05 significance level for testing the null hypothesis of row equals 0, and determine whether there is a significant correlation between the variables. So from the scatter plot in the question, we can observe that our data includes the resting heart rates in BPM of the following values and the reaction times in milliseconds of the following values. And using this data, we can then assign ranks where we rank both sets from lowest to highest. And both sets rank from lowest to highest as follows, which can be observed in this table. And then using the data in the table, we can compute Spearman's rank correlation coefficient of row, starting with taking the sum of our D2 values by adding all of the D2 values together, giving us 572. And then using the formula for Spearman's rank correlation coefficient row, which is equal to 1 minus 6 multiplied by the sum of D quad divided by N multiplied by in parentheses N2 minus 1, which we plug in the values into the formula, and we get our Spearman rank correlation coefficient row equals -1. And then using those values that we have calculated, we can compute the critical value. So for an N equals 12, the critical value of Spearman's row at an alpha of 0.05 and a two-tail test is approximately a row critical of positive or negative 0.591. So in conclusion, since the calculated value of row is equal to negative. 1, which is less than the critical value of -0.591, we reject the null hypothesis. So there is a significant negative correlation between resting heart rate and reaction time. Therefore, the correct answer choice is answer choice B, as row is equal to -1, and there is a significant negative correlation, and all other answer choices are incorrect. I hope you found this video to be helpful. Thank you and goodbye.

��app

Key Concepts

Rank Correlation Coefficient

Null Hypothesis

Critical Values

Watch next