In Exercises 29–32, compute the mean of the data summarized in...

Question

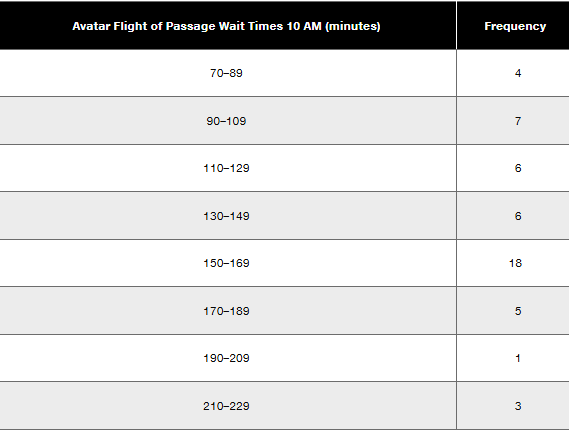
In Exercises 29–32, compute the mean of the data summarized in the frequency distribution. Also, compare the computed means to the actual means obtained by using the original list of data values, which are as follows: (29) 31.4 minutes; (Exercise 30) 140.6 minutes; (Exercise 31) 55.2 years; (Exercise 32) 240.2 seconds.

Table displaying wait times in minutes for the Avatar Flight of Passage ride, with corresponding frequency counts.

Accepted Answer

Welcome back, everyone. In this problem, a study recorded the time in minutes spent on daily exercise by a group of 50 individuals. The collected data was summarized into the following frequency distribution. Compute the mean of the data summarized in the frequency distribution, and then compare the computed mean to the actual mean obtained from the original list of data values, which is 31.2 minutes. A says the computed mean is 34.5 minutes, which is higher than the actual mean of 31.2 minutes. B says it's 31.2 minutes which matches the actual mean exactly. C says it's 28.9 minutes, which is lower than the actual mean of 31.2 minutes, and the D says it's 26.1 minutes, which is lower than the actual mean of 31.2 minutes. Now, if we are going to compute the mean of the summarized data, first we have to ask ourselves, how do we find the mean when we're dealing with class intervals like we have here? Well, to compute the mean, we'll need the midpoint, OK, of each. So if I were to just uh annotate on our table here, we would need the midpoint of each class. And then we would need. And then we would multiply the frequency F by our midpoint that we can call X. So how can we find both of those? Well first, let's start with our midpoints. Recall. Not the midpoint, yeah. Is equal to the lower bound of a class. Plus it's upper bound. Divided by 2, OK. So for example, OK. For the class, for first class from 10 to 19, it's midpoint, OK. Its midpoint would be equal to. 10 Plus 19 the lower plus upper bound divided by 2. 10 + 19 is 29 and 29 divided by 2 equals 14.5. OK? So if we go back to our table here, or midpoint would be 14.5 for the first class. To find a midpoint for the rest, essentially we would do the same thing. So when we add 20 and 29 and divide by 2, we would get 24.5. 30 plus 39 divided by 2 is 34.5. 40 + 49 divided by 2 is 44.5. And I know you probably guessed it, the midpoint for 50 to 59 would be 54.5. Thus No, we multiply the frequency by the midpoint. 6 multiplied by 14.5 is 87. 12 multiplied by 24.5 is 294. 15 multiplied by 34.5 is 517.5. 10 multiplied by 44.5 is 445, and 7 multiplied by 54.5 is 381.5. Now that we have the midpoint and the product of the frequency and the midpoint, the next question is how is this going to help us compute the actual meat? We'll recall as well. That or mean X bar is equal to the sum of the frequencies multiplied by the midpoints divided by the total frequency. If we go back to our table, OK, then notice here that if we add all our frequencies up, our total frequency is 50, OK, which may, and, and that makes sense as well because remember we're told this is a group of 50 individuals and if we sum our frequencies multiplied by the midpoints, then we should get 1,725. OK? So this is our total. So in that case, our our mean is going to be 1,725 divided by 50, which is equal to 34.5 minutes. Thus, the computed mean is 34.5 minutes, and when we compare that with the actual mean of 31.2 minutes, that means the computed mean is higher than the actual mean. Therefore, A is the correct answer. Thanks a lot for watching everyone. I hope this video helped.

��app

Key Concepts

Mean

Frequency Distribution

Comparison of Means

Watch next