In Exercises 17–20, refer to the accompanying table showing re...

Question

In Exercises 17–20, refer to the accompanying table showing results from experiments conducted by researchers Charles R. Honts (Boise State University) and Gordon H. Barland (Department of Defense Polygraph Institute). In each case, it was known whether or not the subject lied, so the table indicates when the polygraph (lie detector) test was correct.

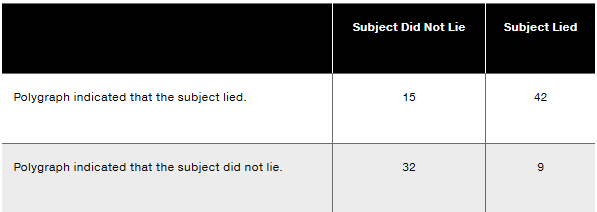
False Negative Find the probability of selecting a subject with a negative polygraph result, given that the subject lied. What would be an unfavorable consequence of this error?

False Negative Find the probability of selecting a subject with a negative polygraph result, given that the subject lied. What would be an unfavorable consequence of this error?

Accepted Answer

Welcome back, everyone. Referred to the table below showing the results from a study where a new allergy detection method was tested. In each case, it was confirmed whether the person actually had the allergy. Find the probability that a randomly selected person received a negative test result given that the person had the allergy. What might be a potential risk of this kind of error. So let's define the context or the goal of the problem. We want to identify a conditional probability. We want to identify the probability of a negative test result, let's call it P of negative. Given That the person had the allergies we're going to say had. So the probability of negative given had. Now, because we're given a table, we don't need to use the formulas for conditional probability. What we can do is simply narrow down the data. It says that we're looking at. The participants who had allergy rights were interested in the last column of the table, and essentially the total number of those who had allergy would be 47, those who tested positive plus 11, those who tested negative, right? So we're going to say 47 + 11. This is the total number of participants in the study who had allergy. And we want to identify those in the same column who tested negative, and those are 11. So now we are ready to valid the probability, which is the ratio between favorable outcomes, those who tested negative, that would be 11, and the total number of participants who had allergy because we're considering a closed subgroup, right, those who had allergy, and the total number corresponds to 47 + 11. Those who tested positive and those who tested negative were the total number of participants who had allergy. And that's it. We want to evaluate the ratio between 11 and 58, which is 0.19. So we can label the first answer to this problem. The probability is 0.19. And now what might be a potential risk of this kind of error? Well, we just need to analyze the definition of this probability. We're looking at a negative test. Given that a participant actually had allergy, right? So. A participant had allergy, but the test indicates that. The participant does not have allergy, right? So what does that mean? Well, it is a. Significant risk. Because The person might be exposed to allergens due to false assurance and suffer health complications, right? Because essentially there are no preservation methods. Or measures taken by a participant thinking that they tested negative, so they basically do not have allergy. So we're going to say because The person Might be He exposed To allergens. Do to False assurance. And suffer health complications, right? So that would be our answer to this problem. Thank you for watching.

��app

Key Concepts

False Negative

Probability

Confusion Matrix

Watch next