Denomination Effect
In Exercises 13–16, use the data in ...

Question

Denomination Effect

In Exercises 13–16, use the data in the following table. In an experiment to study the effects of using four quarters versus a $1 bill, some college students were given four quarters and others were given a $1 bill, and they could either keep the money or spend it on gum. The results are summarized in the table (based on data from “The Denomination Effect,” by Priya Raghubir and Joydeep Srivastava, Journal of Consumer Research, Vol. 36).

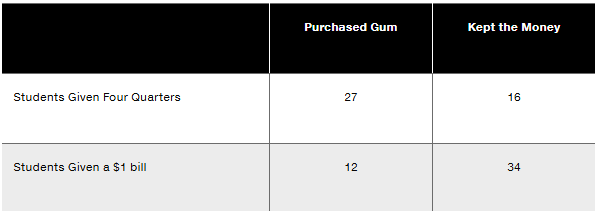
Denomination Effect

a. Find the probability of randomly selecting a student who spent the money, given that the student was given a $1 bill.

Accepted Answer

Hello there. Today we're gonna solve the following practice problem together. So first off, let us read the problem and highlight all the key pieces of information that we need to use in order to solve this problem. A cafe analyzed whether customers tipped based on their payment method. Some customers paid with cash while others paid by card. The results are shown below. So we have a table where the far right column states did not tip, where the left column states left a tip, and on the far left column we have paid with cash and paid by card. So those who paid with cash and left a tip was 33. And those who paid with cash and did not tip was 17. Individuals who paid by card and left a tip was 18, and those who paid by card and did not tip was 42. What is the probability that a randomly selected customer tipped given that the customer paid with card? Awesome. So it appears for this particular problem where ultimately our end goal is we're trying to determine what is the probability that a randomly selected customer left a tip given that this particular customer paid with a card. Awesome. So now that we know that we're trying to determine what this probability value is, let's read off our multiple choice answers to see what our final answer might be. A is 0.05, B is 0.03, C is 0.30, and finally, D is 0.50. Awesome. So our first step that we need to take in order to solve for this particular problem is we need to determine the total number of customers who paid by card. So let us note that we are told by the problem itself that 18 of the customers who paid with card left a tip which will put a capital T in parenthesis to denote tipped. And then we need to add the 42 who did not tip, because we're told from our table, that's given to us by the prom itself that 42 people who paid by card did not leave a tip, so 42, and I'll put it as D did not tip, so D and T did not tip. So that will mean that 18 + 42 will give us a total of 60 individuals who paid with card. Awesome. And now our second step now is we need to determine the number of those specific customers who left a tip, which once again those out of everyone who paid by card, which was a total of 60 people who paid by card, only 18 of them left a tip. Fantastic. Our third step now is we need to recall and apply the conditional probability formula, and as we should recall a note, we're focusing only on the individual customers who used card as their payment method to help us determine the chance of tipping. So that will mean that we need to determine P. Of those who tipped. And paid by card, so paid by card, which are called PBC so paid by card. So we're trying to find the probability P that an individual tipped when they paid with card. And this will be equal to, since we're told that 18 individuals who paid with card did tip, and then we have to divide it by the total number of opportunities or outcomes, which is 60, means 60 total individuals paid by card. So we need to take 18 divided by 60, and when we do that, we will find that it'll be equal to when we round to two decial places, 0.30, and that. Is our final answer. Hooray, we did it, and this corresponds with multiple choice answer letter C, which once again is 0.30. Thank you so much for watching. Hopefully that helped, and I can't wait to see you in the next video. Bye.

��app

Key Concepts

Probability

Conditional Probability